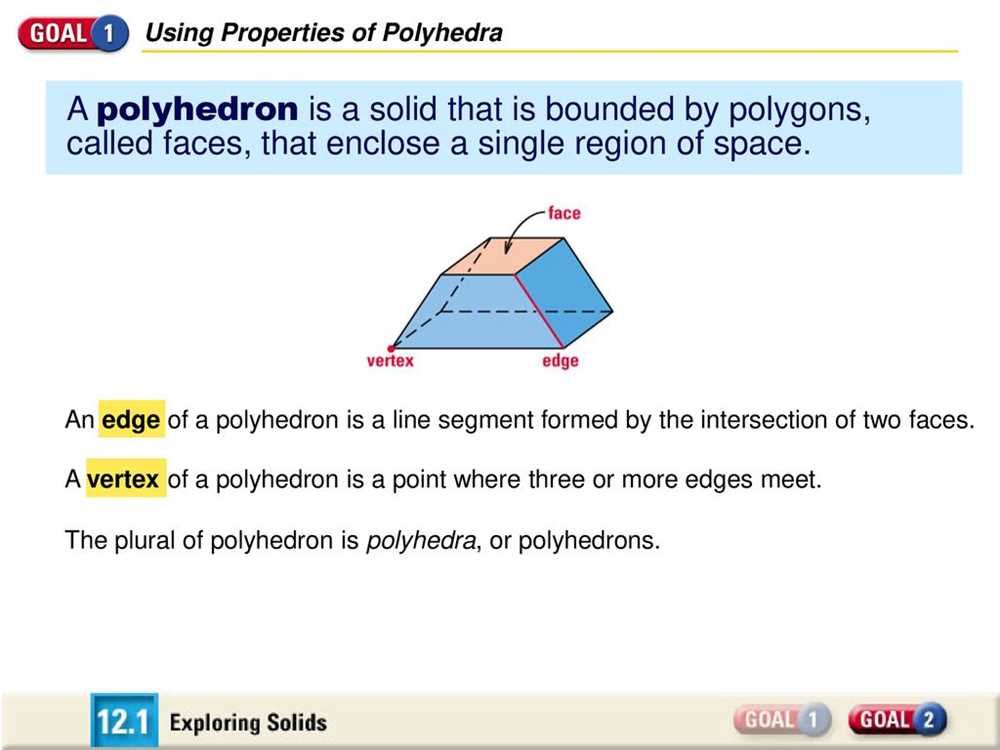
Galxe polyhedra are fascinating geometric objects that have been studied extensively in the field of mathematics and geometry. These unique structures possess a myriad of geometric properties that make them both aesthetically pleasing and intellectually stimulating. In this article, we will delve into the intricacies of galxe polyhedra, exploring their properties, classifications, and applications.
Geometric properties refer to the characteristics and qualities of shapes and figures, such as their edges, faces, vertices, and angles. Galxe polyhedra, in particular, exhibit a remarkable set of properties that distinguish them from other polyhedra. One of the most fascinating aspects of galxe polyhedra is their symmetry. These structures possess a high degree of symmetry, often showcasing rotational symmetry, reflectional symmetry, or a combination of both.
Furthermore, galxe polyhedra are known for their self-dual characteristic, meaning that they can be transformed into an identical structure by interchanging the positions of their vertices and faces. This property adds an extra layer of complexity and beauty to these polyhedra, as it showcases the inherent balance and harmony within their geometric structure.
Another prominent feature of galxe polyhedra is their convexity. Unlike concave polyhedra, which have at least one internal angle greater than 180 degrees, galxe polyhedra are strictly convex, with each internal angle being less than 180 degrees. This property not only gives them a pleasing aesthetic quality but also makes them more suitable for various applications in fields such as architecture, design, and even molecular chemistry.
The Shape and Structure of Galxe Polyhedra
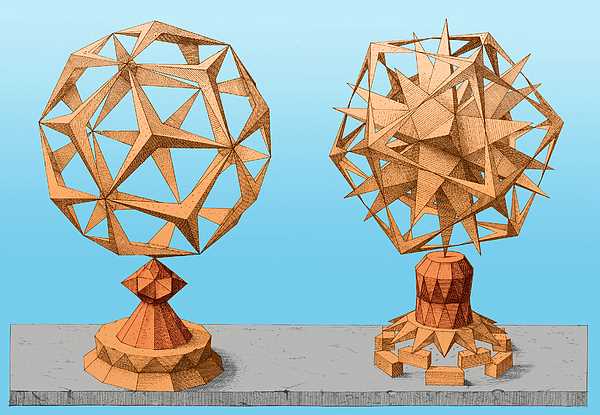
Galxe polyhedra are three-dimensional geometric shapes that consist of a network of polygons. These polygons are connected by edges, creating a closed surface. The shape and structure of galxe polyhedra can vary depending on the number and arrangement of polygons.
One of the defining characteristics of galxe polyhedra is their symmetry. They often exhibit a high degree of symmetry, with regular polygons forming the faces of the polyhedra. This symmetry gives the polyhedra an aesthetically pleasing and balanced appearance.
The vertices of galxe polyhedra are the points where the edges meet. These vertices can be classified based on the number of edges that meet at each vertex. For example, a vertex where three edges meet is called a triangular vertex, while a vertex where four edges meet is called a quadrilateral vertex.
The edges of galxe polyhedra can be straight or curved, depending on the shape of the polygons. Straight edges are a common feature of regular galxe polyhedra, where all the polygons are congruent and the angles and edge lengths are the same.
Another important aspect of the structure of galxe polyhedra is the number of faces, edges, and vertices. Euler’s formula, V – E + F = 2, relates these three quantities for any polyhedron, including galxe polyhedra. This formula can be used to determine the number of faces, edges, or vertices when the other two quantities are known.
In summary, galxe polyhedra are three-dimensional shapes with a network of polygons forming their structure. They exhibit symmetry, have vertices where edges meet, and can have straight or curved edges. The number of faces, edges, and vertices of galxe polyhedra can be determined using Euler’s formula.
The Mathematical Properties of Galxe Polyhedra
Galxe polyhedra are three-dimensional geometric shapes that have unique mathematical properties. These properties govern the relationships between the edges, vertices, and faces of these polyhedra, and are fundamental to understanding their structure and characteristics.
One of the key mathematical properties of Galxe polyhedra is their Euler’s formula, which states that for any convex polyhedron, the number of faces (F), vertices (V), and edges (E) are related by the equation F + V = E + 2. This formula provides a fundamental understanding of the interconnectedness of the different components of a Galxe polyhedron.
Another important mathematical property of Galxe polyhedra is the concept of symmetry. Symmetry refers to the presence of certain transformational properties, such as rotations and reflections, that leave the polyhedron unchanged. Galxe polyhedra can exhibit various types of symmetry, including rotational symmetry, mirror symmetry, and combination symmetry.
Additionally, Galxe polyhedra often exhibit unique geometric properties related to their specific shape and structure. For example, some Galxe polyhedra have equal edge lengths or angles, while others have specific arrangements of vertices that create regular patterns.
Furthermore, the mathematical properties of Galxe polyhedra can be used to classify and categorize them into different types and families. These classifications are based on their symmetries, regularities, or other geometric properties. Understanding the mathematical properties of Galxe polyhedra allows mathematicians and researchers to analyze and compare these shapes, unlocking insights into their inherent structure and behavior.
In conclusion, the mathematical properties of Galxe polyhedra play a crucial role in understanding and studying these geometric shapes. Euler’s formula, symmetry, and other geometric properties provide a foundation for analyzing and classifying Galxe polyhedra, and offer insights into their structure, regularities, and behavior.
Applications and Practical Uses of Galxe Polyhedra
Galxe polyhedra, also known as Galaxy polyhedra, have a variety of applications in various fields. The unique geometric properties of these polyhedra make them suitable for diverse practical uses. Here are some examples:
1. Architecture and Design
Galxe polyhedra can be used in architecture and design to create eye-catching structures. Their intricate shapes and patterns can be incorporated into the design of buildings, bridges, and other architectural elements to add visual interest and complexity. These polyhedra can also be used as the basis for designing innovative furniture and decorative objects.
2. Mathematics and Geometry Education
Galxe polyhedra offer an engaging way to introduce students to the concepts of geometry and mathematical principles. The exploration of these polyhedra can help students understand geometric properties such as faces, edges, and vertices, as well as concepts like symmetry and tessellation. Teachers can use physical models or digital representations of galxe polyhedra to enhance their lessons and make learning more interactive.
| Applications | Practical Uses |
|---|---|
| Architecture and Design | Creating visually interesting structures and decorative objects |
| Mathematics and Geometry Education | Enhancing understanding of geometric properties and concepts |
Question-answer:
What are galxe polyhedra?
Galxe polyhedra are three-dimensional shapes that have a unique geometric property. They are made up of a central core surrounded by interconnected triangular faces, which give them their distinctive appearance.
How do galxe polyhedra relate to other geometric shapes?
Galxe polyhedra are a subclass of polyhedra, which are three-dimensional shapes with flat faces and straight edges. They are distinct from other polyhedra due to their specific geometric properties, including their unusual arrangement of triangular faces.


